Abnormal Return: Definition, Causes, Example

Overview

Investing world is full of numbers, but one key metric separates the good performers from the rest: abnormal return. Abnormal returns, also referred to as excess returns, are a valuable finance metric, especially the stock market. The concept helps investors focus on improving returns and managing risks, whether experienced or new. 

The blog helps explore the meaning of abnormal returns, emphasizing its importance in the investment world. This will further go into its calculating formula and how to calculate it for improved returns on your investment.

What Is An Abnormal Return/Excess Returns?

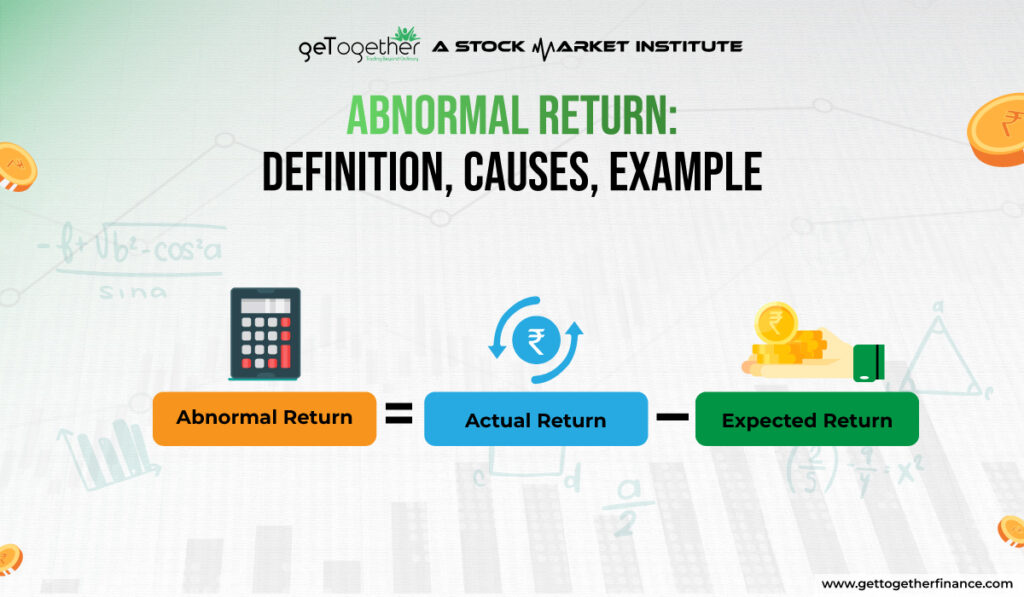
An abnormal return is a term used in finance to define the difference between the actual return of an investment and its expected return. This expected return is typically based on the investment’s risk level and is often calculated using models like the for the market average return.

Here’s a more detailed breakdown:

• Expected Return: The return that an investor anticipates receiving based on historical data and models that consider the investment’s risk and market conditions.
• Actual Return: The return that the investment actually generates over a specific period.
• Abnormal Return/Excess Returns: The difference between the actual return and the expected return. It can be either positive (when the actual return is higher than expected) or negative (when the actual return is lower than expected).

In simpler terms, an AR tells you how much your investment outperformed (positive excess returns) or underperformed (negative excess returns) the market after considering the inherent risk. It’s basically a measure of your stock-picking skills or strategy compared to the baseline market performance.

Formula for Abnormal Return (AR)

Here is the formula for calculating abnormal return (excess return):

Formula of AR  = Actual Return – (Risk-Free Rate + Expected Market Return)

Here:

• Actual Return: The percentage return you earn on your investment over a specific period.
• Risk-Free Rate: The return you would get from a risk-free investment, like government bonds.
• Expected Market Return: This can be estimated using various methods, often based on historical market performance or a benchmark index like the Nifty 50 in India.

How To Calculate Abnormal Return

To calculate the expected return, we can use the Capital Asset Pricing Model (CAPM). The following is the equation for the model:

Er = Rf + β (Rm − Rf)

Here,

• Er = Expected return in the security
• Rf = risk-free rate, generally the rate of a government security or savings deposit rate,
• Beta(β) = risk coefficient of the security or the portfolio in comparison to the market,
• Rm = Return on the market or an appropriate index for the given security such as Nifty 50.

Once we have the expected return, we subtract the same from the actual return to calculate the abnormal return. When the portfolio or security has underperformed expectations, the AR will be negative.

Otherwise, it will be positive or equal to zero, as the case may be.

As per the careful approach, it is better to look at the risk-adjusted return. This is in keeping with the concept of risk tolerance because otherwise, the portfolio manager may differ from the IPS goals and take up highly risky investments to generate abnormal returns/excess returns.

In the case of multiple periods, it may be helpful to look at the standardized returns to see if the portfolio is constantly beating the benchmark.

Example of Abnormal Return/Excess Returns

Let’s update the example with the new values:

• Risk-free rate (RfR) = 4%
• Market return (RmR​) = 12%
• Beta (β) of the portfolio = 1.5
• Beginning value of the portfolio = ₹50,000
• Ending value of the portfolio = ₹60,000

Step 1: Calculate the Expected Return using Capital Asset Pricing Model (CAPM)

The Capital Asset Pricing Model (CAPM) formula is:

Er = Rf + β (Rm − Rf)

Adding in the new values:

Er = 4% + 1.5 × (12% − 4%)

Er = 4% + 1.5 × 8%  

Er = 4% + 12%

Er = 16%

So, the expected return on the portfolio is 16%.

Step 2: Calculate the Actual Return

The actual return (RaR) can be calculated based on the beginning and ending values of the portfolio.

Formula of Actual Return (Ra) = Ending Value − Beginning Value/Beginning Value × 100%

Ra = ₹60,000 − ₹50,000₹/50,000 × 100%  

Ra = ₹10,000/₹50,000 × 100%

Ra​ = 20%

Step 3: Calculate the AR

Now let’s put the value into the excess returns or AR formula to get the final number.

AR = Actual Return (Ra​) − (Risk-Free Rate (Rf​) + Expected Market Return (Rm​))

AR = 20% − (4% + 12%)

AR= 20% − 16%

AR = 4%

In this instance, the AR is 4%. This shows that the portfolio has outperformed the expected return based on its risk level and the overall market return.

Also Read: Binary Options

Importance of Abnormal Return/Excess Returns

Not all investment returns are created equal. While the raw percentage change might seem like the whole story, a deeper metric called “abnormal return” or “excess returns” reveals a more nuanced picture. This metric goes beyond simply tracking gains or losses; it measures how much your investment outperformed or underperformed the market after considering the inherent risk.

Here’s why abnormal returns hold such significance for investors:

Identifying Opportunities

Positive abnormal returns (excess returns) can indicate that an investment has outpaced expectations, potentially signaling an undervalued stock or a smart investment strategy. By assessing companies with consistent positive AR, investors can potentially uncover hidden gems with high growth potential.

Evaluating Performance

Abnormal returns (excess returns) help investors assess the effectiveness of their investment choices or those of a portfolio manager. Studying AR provides valuable insights for making informed investment decisions such as the performance of stocks relative to the market, etc.

Market Efficiency

Abnormal returns (excess returns) can act as a gauge of market efficiency. If a large number of investments consistently generate positive AR, it might suggest the market is not fully capturing all available information, potentially revealing opportunities for skilled investors.

In essence, AR offer a window into the true performance of your investments relative to the market. They empower you to become a more informed investor, identify undervalued assets, evaluate investment strategies, and potentially outperform the market over time.

Conclusion

Understanding abnormal returns empowers you to become a more informed investor in the stock market. It allows you to assess your investment choices, evaluate portfolio managers, and potentially identify opportunities to outperform the market. Remember, AR are just one piece of the puzzle. Consider factors like your risk tolerance and investment goals when making investment decisions. You can also consult with a financial advisor who can provide valuable guidance tailored to your financial circumstances.

FAQs